Mean Sea Level, GPS, and the Geoid
Continued...

The geoid and ellipsoid intersect at the geoid undulations. Undulations result from several phenomena, the most significant of which is the existence of gravitational anomalies caused by the nonhomogeneous nature of the earth. The density of magma in the earth's crust is unevenly distributed. In areas of greater density, it can be significantly higher and, consequently, cooler. Less dense areas are correspondingly lower and hotter. Dense magma exerts a stronger pull, which causes the accumulation of water masses. Not much is known about whether these volumes relocate or how fast they move. If these locations do move, their movement would be at the same pace as other geologic events (i.e., extremely slow).

Precise measurements taken from space are applied to GPS readings. These measurements are based on an ellipsoid surface that is a mathematically generated model of the earth obtained from a three-dimensional Cartesian coordinate system. The GPS receivers can provide only the ellipsoid (geometric) height.

However, most users expect accurate elevation readings that are related to MSL. Consequently, newer GPS devices output orthometric (geoid) height measurements as a product of "behind the scenes" calculations based on a combination of formulas, tables, and matrices that use geographic coordinates as inputs. The appropriate height for the geographic location taken from a coarse or fine DEM matrix is provided instead of a real measurement of the z value (or height). Some receivers use approximations of the geoid height to estimate the orthometric height from the ellipsoid height. Still other units, based on an older technology, provide direct readings of the z value based on the ellipsoid.

A Globally Defined Geoid—Geoid99

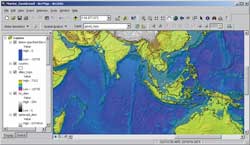This map shows a world with no gravitational anomalies. A hypothetically homogeneous distribution of mass within the earth would change the delineation of oceans and landmasses. This view of Southeast Asia depicts such a speculative shoreline. Note the differences between the current and the theoretical outlines of the world ocean.

A globally defined geoid was required so that GPS receivers could calculate the correct z value needed as a reference surface for a global vertical datum. Geoid99, a model with a submeter level of accuracy, was developed by the National Geodetic Survey. It is used as a zero surface to establish consistent and accurate elevations worldwide. However, despite this impressive level of accuracy, portions of the Geoid99 deviate from MSL due to gravity effects.

The Earth Geodetic Model (EGM96), developed in a collaborative effort by NASA Goddard Space Flight Center, the National Imagery and Mapping Agency (NIMA), and Ohio State University, has been used to compute geoid undulations accurate to better than one meter (except in areas lacking accurate surface gravity data). The values of this surface show at every location how much MSL varies from the ellipsoid used as the reference for GPS elevation readings. In other words, EGM96 shows how uneven the gravitational potential of the ocean's surface really is. The maximum range of the Geoid99 undulations with respect to the WGS84 ellipsoid is 192 meters. The biggest anomaly was discovered southeast of India where the geoid is 105 meters below the ellipsoid, and its highest swelling was observed in eastern Indonesia.

"What If" Simulations

This map shows the hypothetical approximate delineation of landmass and ocean based on a spherically-shaped earth, which assumes that the current relief would be preserved.

How would continental coastlines change if there were no gravitational anomalies? The short answer would be that they would appear the same as if the earth were an ellipsoid. The biggest undulations are concentrated in the northern Indian Ocean and within the Indonesian Archipelago. Consequently, areas currently covered by the Arafura Sea and Carpenteria Bay, both north of Australia, would emerge. Conversely other areas, such as the lowlands formed by the deltas of some of the main South Asian rivers such as the Ganges and Brahmaputra as well as those of Indus, Irrawaddy, and Mekong, would sink.

As an intellectual exercise, imagine for a moment that the earth has the geometrically perfect shape of a spheroid and then an ellipsoid. To visualize these "what if" scenarios, a computer simulation was generated using rasters that represented distances to the earth's center and were generated with the Raster Calculator in the ArcGIS Spatial Analyst extension. A standard digital elevation model for the globe was modified by removing the gravitational anomalies identified by EGM96. These geometrically correct representations of a spheroid and ellipsoid were used to produce GIS simulations called the SPHEROID DEM and ELLIPSOID DEM. The accompanying illustrations show the effects these shapes, as well as other scenarios, have on the distribution of landmass and ocean.

SPHEROID DEM

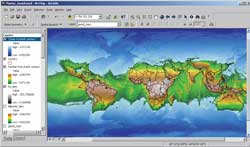What if the earth actually stood still? The data approximates a theoretical elevation model with the elevations relative to the distance from the earth's center. The GPS refers its direct elevation readings to this shape. The cell values of the above ellipsoid_dem grid are the direct readings of GPS. The displayed GPS readings are based on the added gravitational correction as the shape of the geoid is based on gravitational potential (both positive and negative).

The SPHEROID DEM was created based on the assumption that the earth's shape, or more precisely, the shape of the earth's surface as represented by MSL, is spherical. In other words, the globe's radius connecting the center of mass with the hypothetical equipotential gravitational surface is identical everywhere on the planet. The radius was set to 6,367,473 meters, which is the distance to the earth's center from the ellipsoidal reference surface at 45 degrees latitude.

This change in geometry to a spheroid geometry with its altered gravity would cause the global ocean to change. The polar zones would be relatively farther from the center of the earth, and these new higher altitudes would force seawater toward the equator. By the same token, the equatorial region would be relatively closer to the earth's center and would be more strongly affected by gravity. Increased gravitational force in the equatorial zone would pull oceanic water toward the equator and form a global equatorial ocean.

Continued on page 3