Mean Sea Level, GPS, and the Geoid
By Witold Fraczek, Esri Applications Prototype Lab

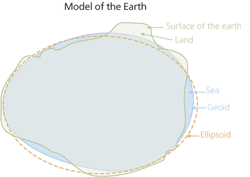The geoid approximates mean sea level. The shape of the ellipsoid was calculated based on the hypothetical equipotential gravitational surface. A significant difference exists between this mathematical model and the real object. However, even the most mathematically sophisticated geoid can only approximate the real shape of the earth.

Frequently research and technology endeavors have unforeseen but positive outcomes. When European explorers set out to find a shortcut to India, they discovered the New World. When a staphylococci bacteria culture was mistakenly contaminated with a common mold, the clear area between the mold and the bacterial colony led to the conclusion that the mold, Penicillin notatum, produced a compound that inhibited the growth of bacteria. This chance discovery led to the development of the antibiotic penicillin.

That the earth does not have a geometrically perfect shape is well established, and the geoid is used to describe the unique and irregular shape of the earth. However, only recently have the more substantial irregularities in the surface created by the global mean sea level (MSL) been observed. These irregularities are an order of magnitude greater than experts had predicted. Controlled by the gravitational potential of the earth, these irregularities form very gentle but massive "hills" and "valleys." This astonishing finding was made possible through the use of GPS, a technology designed by the United States Department of Defense to revolutionize navigation for the U.S. Navy and Air Force. GPS has done that—and a lot more.

What Is Mean Sea Level?

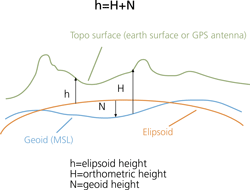The accuracy of GPS height measurements depends on several factors but the most crucial one is the "imperfection" of the earth's shape. Height can be measured in two ways. The GPS uses height (h) above the reference ellipsoid that approximates the earth's surface. The traditional, orthometric height (H) is the height above an imaginary surface called the geoid, which is determined by the earth's gravity and approximated by MSL. The signed difference between the two heights—the difference between the ellipsoid and geoid—is the geoid height (N). The figure above shows the relationships between the different models and explains the reasons why the two hardly ever match spatially.

For generations, the only way to express topographic or bathymetric elevation was to relate it to sea level. Geodesists once believed that the sea was in balance with the earth's gravity and formed a perfectly regular figure. MSL is usually described as a tidal datum that is the arithmetic mean of hourly water elevations observed over a specific 19-year cycle. This definition averages out tidal highs and lows caused by the changing effects of the gravitational forces from the moon and sun.

MSL is defined as the zero elevation for a local area. The zero surface referenced by elevation is called a vertical datum. Unfortunately for mapmakers, sea level is not a simple surface. Since the sea surface conforms to the earth's gravitational field, MSL also has slight hills and valleys that are similar to the land surface but much smoother. However, zero elevation as defined by Spain is not the same zero elevation defined by Canada, which is why locally defined vertical datums differ from each other.

The MSL surface is in a state of gravitational equilibrium. It can be regarded as extending under the continents and is a close approximation of the geoid. By definition, the geoid describes the irregular shape of the earth and is the true zero surface for measuring elevations. Because the geoid surface cannot be directly observed, heights above or below the geoid surface can't be directly measured and are inferred by making gravity measurements and modeling the surface mathematically. Previously, there was no way to accurately measure the geoid so it was roughly approximated by MSL. Although for practical purposes, at the coastline the geoid and MSL surfaces are assumed to be essentially the same, at some spots the geoid can actually differ from MSL by several meters.

Differing Measurements

GPS has transformed how altitude at any spot is measured. GPS uses an ellipsoid coordinate system for both its horizontal and vertical datums. An ellipsoid—or flattened sphere—is used to represent the geometric model of the earth.

The surface of global undulations was calculated based on altimetric observations and very precise (up to two centimeters) measurements taken from the TOPEX/POSEIDON satellite. This data was represented in the Earth Geodetic Model (EGM96), which is also referred to as the spherical harmonic model of the earth's gravitational potential.

Conceptually, this precisely calculated ellipsoid, called an oblate ellipsoid of revolution, was intended to replicate the MSL as the main geodetic reference or vertical datum. If this ellipsoid vertical datum is used, height above the ellipsoid will not be the same as MSL and direct elevation readings for most locations will be embarrassingly off. This is caused, in part, because the GPS definition of altitude does not refer to MSL, but rather to a gravitational surface called the reference ellipsoid. Because the reference ellipsoid was intended to closely approximate the MSL, it was surprising when the two figures differed greatly.

The TOPEX/POSEIDON satellite, launched in 1992, was specifically designed to perform very precise altimetric observations. These measurements have demonstrated that neither human error nor GPS inaccuracies are responsible for the sometimes substantial discrepancies between ellipsoid and MSL measurements. In fact, the three-dimensional surface created by the earth's sea level is not geometrically correct, and its significant irregularities could not be mathematically calculated; this explains the difference between the ellipsoid-based GPS elevation readings and elevations shown on accurate topographic maps.

A brief examination of elevation readings for Esri headquarters in Redlands, California, demonstrates these differences. The campus elevation is shown on topographic quadrangle maps and high-resolution digital elevation models (DEMs) for the area as approximately 400 meters above MSL. However, a precise, nonadjusted GPS reading for the same location typically shows the elevation as 368 meters.

The map shows the areas of the globe that would have a sea level below the theoretical surface of the WGS84 ellipsoid, or the theoretical and geometrically correct sea level (shown in blue). The sharp contrast between the blue and green indicates where the ellipsoid and geoid intersect. With the continents displayed as opaque, the remaining area covered by water reveals where sea level is actually at zero elevation relative to the WGS84 ellipsoid.

Why is there a 32-meter difference? The GPS receiver uses a theoretical sea level estimated by a World Geodetic System (WGS84) ellipsoid, which does not perfectly follow the theoretical MSL. The MSL, approximated by an ellipsoid, is related to gravity or the center of mass of the earth. Discrepancies between a WGS84 ellipsoid, and the geoid vary with location. To continue with this example, elevation readings for Yucaipa, a city located less than 10 miles east of Redlands, differ by 31.5 meters.

Continued on page 2